| 1. Inputs | |
| Ideally-expanded Mach Number [M] | |
| Working Fluid | |
| Gas Constant [R] (J/Kg.K) | |
| Specific Heat Ratio [ɣ] | |
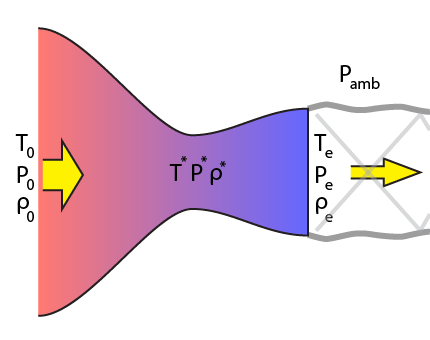
| Stagnation Pressure [P0] (Pa) | |
| Stagnation Temperature [T0] (C) | |
| Stagnation Density [ρ0] (Kg/m3) | |
| Ambient Pressure [Pamb] (Pa) | |
| Throat Diameter [D*] (mm) | |
| "Stagnation" Pipe Diameter [D0] (mm) | |
| 2.1. Isentropic Flow Equation Ratios: | |
| Nozzle Pressure Ratio (NPR) [P0/P] | |
| Nozzle Temperature Ratio (NTR) [T0/T] | |
| Density Ratio [ρ0/ρ] | |
| Throat Pressure Ratio [P*/P] | |
| Throat Temperature Ratio [T*/T] | |
| Throat Density Ratio [ρ*/ρ] | |
| Exit Area Ratio [A/A*] | |
| 2.2. Physical Nozzle Conditions: | |
| 2.2.1. Throat Conditions: | |
| Throat Temperature [T*] (C) | |
| Throat Pressure [P*] (Pa) | |
| Throat Density [ρ*] (Kg/m3) | |
| Speed of Sound at Throat [a*] (m/s) | |
| 2.2.2. Exit Conditions: | |
| Exit Temperature [Te] (C) | |
| Exit Pressure [Pe] (Pa) | |
| Exit Density [ρe] (Kg/m3) | |
| Speed of Sound at Exit [ae] (m/s) | |
| Exit Velocity [ve] (m/s) | |
| 2.2.3. Inlet Conditions: | |
| Inlet Temperature [T0] (C) | |
| Inlet Pressure [P0] (Pa) | |
| Inlet Density [ρ0] (Kg/m3) | |
| Speed of Sound at Inlet [a0] (m/s) | |
| Inlet Velocity [v0] (m/s) | |
| Inlet Mach Number [M0] | |
| 2.2.4. Physical Dimensions: | |
| Nozzle Throat Area [A*] (mm2) | |
| Nozzle Exit Area [Ae] (mm2) | |
| Nozzle Exit Diameter [De] (mm) | |
| 2.3. Nozzle Flow Properties: | |
| Mass Flow Rate [ṁ] (g/s) | |
| Thrust (N) | |
| Compressor Power (W) | |
| Flow Power (W) |
 |
| |